THE ELECTRONIC JOURNAL OF COMBINATORICS (ed. June 2005), DS #5.

Venn Diagram Survey
Diagrams Made from Triangles

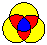
There is a simple symmetric 5-Venn diagram in which each curve is a triangle; see this figure, redrawn from Grünbaum [Gr92b]. Grünbaum [Gr84b] asked whether there was a 6-Venn diagram made from triangles. This question was recently answered in the affirmative by Jeremy Carroll [Car00] - his first example is shown below. The diagrams on this page are all simple. He has subsequently discovered that there are exactly 126 different 6-Venn diagrams that can be drawn where each curve is a triangle.

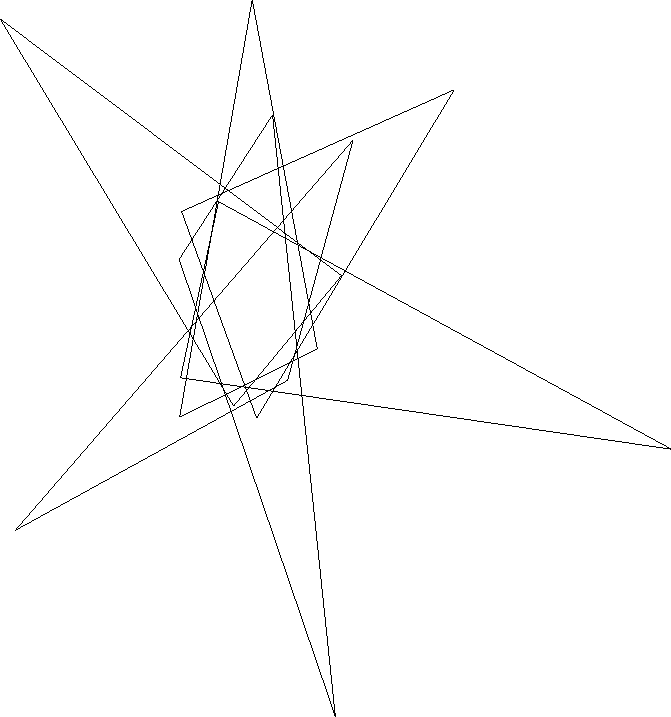
Below is a table of coordinates for the six triangles.

Below is another nicer example (this jpeg image courtesy of Jeremy Carroll). See his web page [JC] for further examples like this.

• Larger version of first example.
• An even larger version of the first example.
• First example with coloured triangles.
• Larger version of first example with bipartite colouring.
• Clipped version of bipartite colouring.
• Black and white version of second example (gif courtesy of Jeremy Carroll).
• Another Coloured version the second example (gif courtesy of Jeremy Carroll).

THE ELECTRONIC JOURNAL OF COMBINATORICS (ed. June 2005), DS #5.